Page 45 - 4560

N   cos    0,5sin  P ;

Q   sin    0,5cos  P ;
M  PR 1 cos  0,5sin  .
  
By getting hold of formulas build diagrams N, Q and M (fig. 4.2,
b).
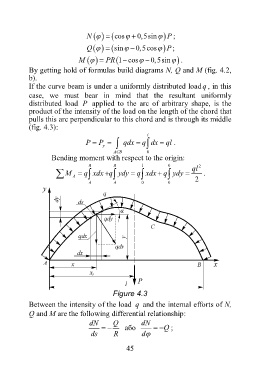
If the curve beam is under a uniformly distributed load q , in this
case, we must bear in mind that the resultant uniformly
distributed load P applied to the arc of arbitrary shape, is the
product of the intensity of the load on the length of the chord that
pulls this arc perpendicular to this chord and is through its middle
(fig. 4.3):
l
P  P   qdx  q dx  ql .

y
ACB 0
Bending moment with respect to the origin:
B B l 0 ql 2
 M  q xdx q ydy   q xdx q ydy   .


А
A A 0 0 2

Figure 4.3

Between the intensity of the load q and the internal efforts of N,
Q and M are the following differential relationship:
dN Q dN
  або   Q ;
ds R d

45