Page 26 - 4560

   . (2.5)
nm mn

2.3 Kastyliano’s Theorem

Moving the point of application of the generalized force in
the direction of its action is partial derivative of the expression
for the potential energy of deformation of this force.
 U
  . (2.6)
p
 P
Substituting expression (2.2) into the formula (2.6) and
use the parameter differentiation rule, we obtain
N dx N M dx M M dx M M dx M y
y
p 
  x x   x x   z z   . (2.7)
l EF  P l EJ k  P l EJ z  P l GJ y  P
For example, in the flat bending problems deflection at the
point of application of concentrated force P is
i
 U M dx M
y    z z , (2.8)
i
 P EJ  P
i l z i
and the angle of rotation where the applied concentrated moment
M will be
i
 U M dx M
    z z . (2.9)
i
 M EJ  M
i l z i
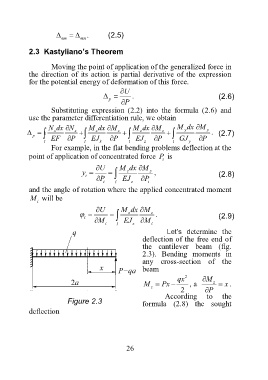
Let’s determine the
deflection of the free end of
the cantilever beam (fig.
2.3). Bending moments in
any cross-section of the
beam
qx 2  M
M  Px  , а z  x .
z 2  P
According to the
Figure 2.3 formula (2.8) the sought
deflection

26