Page 21 - 4549

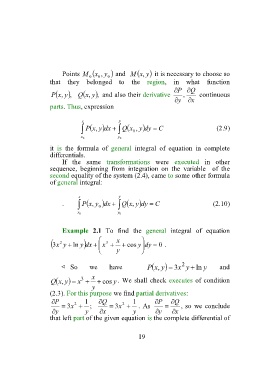
Points M  , yx  and  yxM ,  it is necessary to choose so
0 0 0
that they belonged to the region, in what function
 P  Q
P  , yx , Q  , yx , and also their derivative , continuous
y  x 
parts. Thus, expression

x y

P  yx, dx   Q x , y dy  С (2.9)
0
x 0 y 0

it is the formula of general integral of equation in complete
differentials.
If the same transformations were executed in other
sequence, beginning from integration on the variable of the
second equality of the system (2.4), came to some other formula
of general integral:

x y

. P  yx, 0 dx   Q  yx, dy  С (2.10)
x 0 y 0

Example 2.1 To find the general integral of equation
 x 
3x 2 y  ln y dx   x 3   cos dyy   0 .

 y 

2
 So we have P  yx,  3 x y ln y and
Q  yx,  x  3 x  cos y . We shall check executes of condition
y
(2.3). For this purpose we find partial derivatives:
 P 2 1  Q 2 1  P  Q
 x 3  ;  x 3  . As  , so we conclude
y  y x  y y  x 
that left part of the given equation is the complete differential of

19