Page 28 - 4461

3.2 Reversible Processes. Entropy
A heat engine performing a Carnot’s cycle satisfies the relation:
q T q q
1 2  1 1 , or 1  2 , (3.3)
q T T T
1 2 1 2
where q 1 is the heat absorbed by the engine at higher temperature T 1 and q 2 is the heat
rejected by the engine at lower temperature T 2. As the sign of q 2 is negative, the relation
may be written as
q q q
1  2 , or   0 (3.4)
T T T
1 2
q
This equation states that the sum of the algebraic quantities is zero for a Carnot’s
T
cycle. From this relation Clausius deduced important of thermodynamic function
“entropy”, which proved to be great importance in the development of the
thermodynamics.
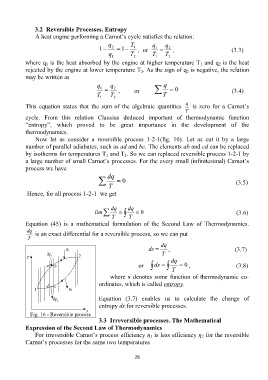
Now let as consider a reversible process 1-2-1(fig. 10). Let as cut it by a large
number of parallel adiabates, such as ad and bc. The elements ab and cd can be replaced
by isotherms for temperatures T 1 and T 2. So we can replaced reversible process 1-2-1 by
a large number of small Carnot’s processes. For the every small (infinitesimal) Carnot’s
process we have
dq
  0 (3.5)
T
Hence, for all process 1-2-1 we get

dq dq
lim     0 (3.6)
T T
Equation (45) is a mathematical formulation of the Second Law of Thermodynamics.
dq
is an exact differential for a reversible process, so we can put
T
dq
q 1 ds  , (3.7)
q  1 T
T 2 dq
a b or ds    0 , (3.8)

T
where s denotes some function of thermodynamic co-
ordinates, which is called entropy.
1 d c q 2

q  Equation (3.7) enables us to calculate the change of
2
entropy ds for reversible processes.
s
Fig. 16 - Reversible process
3.3 Irreversible processes. The Mathematical
Expression of the Second Law of Thermodynamics
For irreversible Carnot’s process efficiency η 1 is less efficiency η 2 for the reversible
Carnot’s processes for the same two temperatures

28