Page 24 - 4461

Change of the entropy:
V
 s  C pm ln 2 (2.8)
P
V
1
From the equation l  T ( R  T ) we can understand what is gas constant R – it is the
P 2 1
work of 1 kg of a gas in the process at the constant pressure when the temperature is
changed through 1K (1˚C).

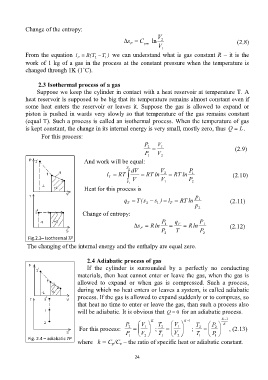
2.3 Isothermal process of a gas
Suppose we keep the cylinder in contact with a heat reservoir at temperature T. A
heat reservoir is supposed to be big that its temperature remains almost constant even if
some heat enters the reservoir or leaves it. Suppose the gas is allowed to expand or
piston is pushed in wards very slowly so that temperature of the gas remains constant
(equal T). Such a process is called an isothermal process. When the temperature of gas
L
is kept constant, the change in its internal energy is very small, mostly zero, thus Q  .
For this process:
P V
2  1 (2.9)
P V
1 2
And work will be equal:
2 V dV V P
l  RT  RT ln 2  RT ln 1
T  V V P (2.10)
1 V 1 2
Heat for this process is
p
q  s ( T 2  s 1 )  l  RT ln 1 (2.11)
T
T
p
2
Change of entropy:
P q P
s  P R ln 1  T  R ln 1 (2.12)
P 2 T P 2
Fig.2.3– Isothermal TP

The changing of the internal energy and the enthalpy are equal zero.

2.4 Adiabatic process of gas
If the cylinder is surrounded by a perfectly no conducting
materials, then heat cannot enter or leave the gas, when the gas is
allowed to expand or when gas is compressed. Such a process,
during which no heat enters or leaves a system, is called adiabatic
process. If the gas is allowed to expand suddenly or to compress, so
that heat no time to enter or leave the gas, than such a process also
will be adiabatic. It is obvious that Q  0 for an adiabatic process.
K 1
K K 1 
P  V  T  V  T  P  K
For this process: 2   1  ; 2   1  ; 2   2  , (2.13)






P 1  V 2  T 1  V 2  T 1  P 1 
Fig. 2.4 – adiabatic TP
where k = C p/C v – the ratio of specific heat or adiabatic constant.

24