Page 20 - 4263

Elements of Vector Algebra

Example 3.3.


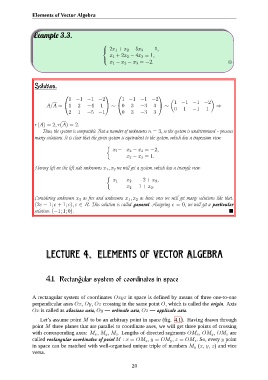
 2x 1 + x 2 − 5x 3 = −1,
x 1 + 2x 2 − 4x 3 = 1,

x 1 − x 2 − x 3 = −2. ,

Solution.

   
1 −1 −1 −2 1 −1 −1 −2 ( )
1 −1 −1 −2
A/A =  1 2 −4 1  ∼  0 3 −3 3  ∼ ⇒
0 1 −1 1
2 1 −5 −1 0 3 −3 3

r(A) = 2, r(A) = 2.
Thus, the system is compatible. But a number of unknowns n = 3, so the system is undetermined — posseses
many solutions. It is clear that the given system is equivalent to the system, which has a trapezium view:

{
x 1 − x 2 − x 3 = −2,
x 2 − x 3 = 1.

Having left on the left side unknowns x 1 , x 2 we will get a system, which has a triangle view:

{
x 1 − x 2 = −2 + x 3 ,
x 2 = 1 + x 3 .

Considering unknown x 3 as free and unknowns x 1 , x 2 as basic ones we will get many solutions like that:
(2c − 1; c + 1; c), c ∈ R. This solution is called general. Assigning c = 0, we will get a particular
solution: (−1; 1; 0).

Lecture 4. Elements of Vector Algebra

4.1. Rectangular system of coordinates in space

A rectangular system of coordinates Oxyz in space is defined by means of three one-to-one
perpendicular axes Ox, Oy, Oz crossing in the same point O, which is called the origin. Axis
Ox is called as abscissa axis, Oy — ordinate axis, Oz — applicate axis.

Let’s assume point M to be an arbitrary point in space (fig. 4.1). Having drawn through
point M three planes that are parallel to coordinate axes, we will get three points of crossing
with corresponding axes: M x , M y , M z . Lengths of directed segments OM x , OM y , OM z are
called rectangular coordinates of point M : x = OM x , y = OM y , z = OM z . So, every y point
in space can be matched with well-organised unique triple of numbers M y (x, y, z) and vice
versa.

20