Page 15 - 4263

Rank of a matrix

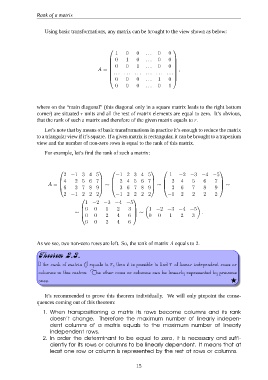
Using basic transformations, any matrix can be brought to the view shown as below:

 
1 0 0 . . . 0 0
0 1 0 . . . 0 0
 
 
 0 0 1 . . . 0 0 
A =   ,
 . . . . . . . . . . . . . . . . . . 
 
 0 0 0 . . . 1 0 
0 0 0 . . . 0 1

where on the “main diagonal” (this diagonal only in a square matrix leads to the right bottom
corner) are situated r units and all the rest of matrix elements are equal to zero. It’s obvious,
that the rank of such a matrix and therefore of the given matrix equals to r.
Let’s note that by means of basic transformations in practice it’s enough to reduce the matrix
to a triangular view if it’s square. If a given matrix is rectangular, it can be brought to a trapezium
view and the number of non-zero rows is equal to the rank of this matrix.

For example, let’s find the rank of such a matrix:

     
2 −1 3 4 5 −1 2 3 4 5 1 −2 −3 −4 −5
 4 −2 5 6 7   −2 4 5 6 7   −2 4 5 6 7 
A =   ∼   ∼   ∼
 6 −3 7 8 9   −3 6 7 8 9   −3 6 7 8 9 
2 −1 2 2 2 −1 2 2 2 2 −1 2 2 2 2
 
1 −2 −3 −4 −5
( )
 0 0 1 2 3  1 −2 −3 −4 −5
∼   ∼ .
 0 0 2 4 6  0 0 1 2 3
0 0 2 4 6

As we see, two non-zero rows are left. So, the rank of matrix A equals to 2.

Theorem 2.3.
If the rank of matrix A equals to r, then it is possible to find r of linear independent rows or
columns in this matrix. The other rows or columns can be linearly represented by previous
ones. ⋆

It’s recommended to prove this theorem individually. We will only pinpoint the conse-
quences coming out of this theorem:

1. When transpositioning a matrix its rows become columns and its rank
doesn’t change. Therefore the maximum number of linearly indepen-
dent columns of a matrix equals to the maximum number of linearly
independent rows.
2. In order the determinant to be equal to zero, it is necessary and suffi-
ciently for its rows or columns to be linearly dependent. It means that at
least one row or column is represented by the rest of rows or columns.

15