Page 19 - 4263

Gauss’ method of successive exception of unknowns

(3.5) has the only solution, so it is compatible and determined. If the matrix we got, has a
trapezium shape, then the system (3.5) is compatible and nondetermined, so that it has many
solutions. After that, we are able to find a solution of the system (3.5) directly.

Remark 3.1. While performing basic transformations we got all the coefficients
of any equation equal to zero and at the same time a free term of that equation
doesn’t equal to zero, then such a system is noncompatible.

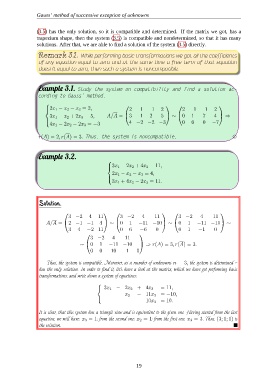
Example 3.1. Study the system on compatibility and find a solution ac-
cording to Gauss’ method.

    
2x 1 − x 2 − x 3 = 2, 2 −1 −1 2 2 −1 −1 2

3x 1 − x 2 + 2x 3 = 5, A/A =  3 −1 2 5  ∼  0 1 7 4  ⇒

 4 −2 −2 −3 0 0 0 −7
4x 1 − 2x 2 − 2x 3 = −3
r(A) = 2, r(A) = 3. Thus, the system is noncompatible. ,

Example 3.2.

3x 1 − 2x 2 + 4x 3 = 11,

2x 1 − x 2 − x 3 = 4,


3x 1 + 4x 2 − 2x 3 = 11.

Solution.

     
3 −2 4 11 3 −2 4 11 3 −2 4 11
A/A =  2 −1 −1 4  ∼  0 1 −11 −10  ∼  0 1 −11 −10  ∼
3 4 −2 11 0 6 −6 0 0 1 −1 0
 
3 −2 4 11
∼  0 1 −11 −10  ⇒ r(A) = 3, r(A) = 3.
0 0 10 1 0

Thus, the system is compatible. Moreover, as a number of unknowns n = 3, the system is determined —
has the only solution. In order to find it, let’s have a look at the matrix, which we have got performing basic
transformations, and write down a system of equations:

= 11,
 3x 1 − 2x 2 + 4x 3
x 2 − 11x 3 = −10,

10x 3 = 10.

It is clear, that this system has a triangle view and is equivalent to the given one. Having started from the last
equation, we will have: x 3 = 1; from the second one: x 2 = 1; from the first one: x 3 = 3. Thus, (3; 1; 1) is
the solution.

19