Page 80 - 4234

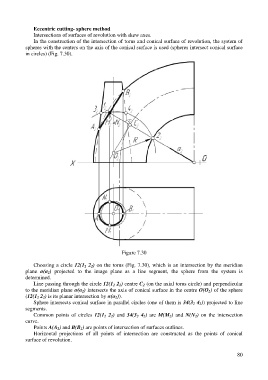
Eccentric cutting- sphere method
Intersections of surfaces of revolution with skew axes.
In the construction of the intersection of torus and conical surface of revolution, the system of
spheres with the centers on the axis of the conical surface is used (spheres intersect conical surface
in circles) (Fig. 7.30).

Figure 7.30

Choosing a circle 12(1 2 2 2) on the torus (Fig. 7.30), which is an intersection by the meridian
plane α(α 2) projected to the image plane as a line segment, the sphere from the system is
determined.
Line passing through the circle 12(1 2 2 2) centre C 2 (on the axial torus circle) and perpendicular
to the meridian plane α(α 2) intersects the axis of conical surface in the centre O(O 2) of the sphere
(12(1 2 2 2) is its planar intersection by α(α 2)).
Sphere intersects conical surface in parallel circles (one of them is 34(3 2 4 2)) projected to line
segments.
Common points of circles 12(1 2 2 2) and 34(3 2 4 2) are M(M 2) and N(N 2) on the intersection
curve.
Points A(A 2) and B(B 2) are points of intersection of surfaces outlines.
Horizontal projections of all points of intersection are constructed as the points of conical
surface of revolution.

80