Page 16 - 4167

 

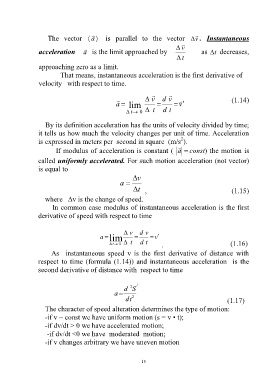
The vector  a is parallel to the vector  . Instantaneous
v

  v
acceleration a is the limit approached by as t decreases,
 t
approaching zero as a limit.

That means, instantaneous acceleration is the first derivative of

velocity with respect to time.

 



v
v
d
(1.14)
v


a 
lim  t d t
 t 0

By its definition acceleration has the units of velocity divided by time;
it tells us how much the velocity changes per unit of time. Acceleration
2
is expressed in meters per second in square (m/s ).

If modulus of acceleration is constant ( a  const) the motion is
called uniformly accelerated. For such motion acceleration (not vector)
is equal to
 v
a 
t 
, (1.15)
where ∆v is the change of speed.

In common case modulus of instantaneous acceleration is the first
derivative of speed with respect to time

 v d v
a  lim   v
 t 0  t d t . (1.16)
As instantaneous speed v is the first derivative of distance with

respect to time (formula (1.14)) and instantaneous acceleration is the
second derivative of distance with respect to time

d 2 S /
a 2
t d
(1.17)
The character of speed alteration determines the type of motion:
-if v ~ const we have uniform motion (s = v • t);
-if dv/dt > 0 we have accelerated motion;

-if dv/dt <0 we have moderated motion;
-if v changes arbitrary we have uneven motion

15