Page 19 - 4167

  
 v  v  v 
 a   1  2
t  t  t  . (1.26)

  v
Instantaneous acceleration a is the limit approached by as
 t
t  decreases, approaching zero as a limit i.e. the first derivative of

velocity with respect to time    

  v  v  v v d v d
a  lim  lim 1  lim 2  1  2
 t 0 t   t 0 t   t 0 t  dt dt . (1.27)


v d
The augend 1
dt
characterizes change
of velocity by

magnitude, the

v d
addend 2
dt
characterizes the
change of velocity
by direction. That is
why , there are

really two
accelerations. And
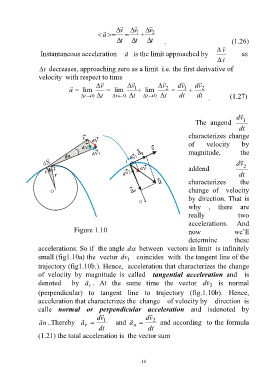
Figure 1.10 now we’ll

determine these
accelerations. So if the angle d between vectors in limit is infinitely
small (fig1.10a) the vector dv coincides with the tangent line of the
1
trajectory (fig1.10b.). Hence, acceleration that characterizes the change
of velocity by magnitude is called tangential acceleration and is
 
v
denoted by a . At the same time the vector d is normal
2

(perpendicular) to tangent line to trajectory (fig.1.10b). Hence,
acceleration that characterizes the change of velocity by direction is
calle normal or perpendicular acceleration and isdenoted by


  v d 1  v d 2
a n..Thereby a  and a  and according to the formula

n
dt dt
(1.21) the total acceleration is the vector sum

18