Page 15 - 4167

According to the definition of displacement quotient obtained
by dividing the vector of displacement by time t is vector l and this
vector is called velocity 
 r
v  . (1.10)
 t
Average velocity  v of point's motion in time interval ∆t is the vector
namely quotient obtained by dividing the vector of displacement by

time 
 r 
v   . (1.11)
t 
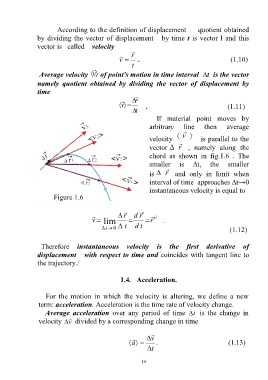
If material point moves by
arbitrary  then average
line
 v 
velocity  is parallel to the
vector  r , namely along the
chord as shown in fig.1.6 . The

smaller is ∆t, the smaller

is  r and only in limit when

interval of time approaches ∆t→0
instantaneous velocity is equal to
Figure 1.6

 
  r d r  /
v  lim   r .
 t 0  t t d (1.12)

Therefore instantaneous velocity is the first derivative of
displacement with respect to time and coincides with tangent line to
the trajectory./

1.4. Acceleration.

For the motion in which the velocity is altering, we define a new

term: acceleration. Acceleration is the time rate of velocity change.
Average acceleration over any period of time t is the change in

velocity v divided by a corresponding change in time


  v
a  . (1.13)
t 

14