Page 101 - 4167

F
x  (8.1)
k
where к is known as the force constant.

Hook's law states that the deformation of
an elastic body is directly proportional to the
applied force.

Whenever an elastic body is distorted by a
force applied by some agent, the body exerts an
equal and opposite force on the agent. Besides,

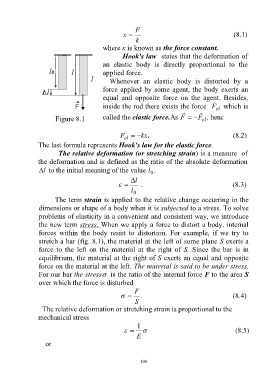
inside the rod there exists the force F which is
el
 
Figure 8.1 called the elastic force.As F   F , henc
el

F   kx. (8.2)
el
The last formula represents Hook's law for the elastic force.

The relative deformation (or stretching strain) is a measure of
the deformation and is defined as the ratio of the absolute deformation
l  to the initial meaning of the value l .
0
l 
 . (8.3)
l 0

The term strain is applied to the relative change occurring in the
dimensions or shape of a body when it is subjected to a stress. To solve
problems of elasticity in a convenient and consistent way, we introduce
the new term stress. When we apply a force to distort a body, internal

forces within the body resist to distortion. For example, if we try to
stretch a bar (fig. 8.1), the material at the left of some plane S exerts a
force to the left on the material at the right of S. Since the bar is in

equilibrium, the material at the right of S exerts an equal and opposite
force on the material at the left. The material is said to be under stress.
For our bar the stress is the ratio of the internal force F to the area S

over which the force is disturbed
F
  . (8.4)
S
The relative deformation or stretching strain is proportional to the
mechanical stress

1
   (8.5)
E
or

100