Page 53 - 6637

Solved Problems

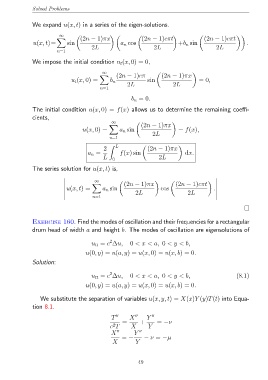
We expand u(x, t) in a series of the eigen-solutions.

∞
X (2n − 1)πx (2n − 1)cπt (2n − 1)cπt
u(x, t)= sin a cos +b sin .
n
n
2L 2L 2L
n=1
We impose the initial condition u (x, 0) = 0,
t
∞
X (2n − 1)cπ (2n − 1)πx
u (x, 0) = b n sin = 0,
t
2L 2L
n=1
b = 0.
n
The initial condition u(x, 0) = f(x) allows us to determine the remaining coeffi-
cients,
∞
X (2n − 1)πx
u(x, 0) = a sin = f(x),
n
2L
n=1
2 Z L (2n − 1)πx
a = f(x) sin dx.
n
L 0 2L
The series solution for u(x, t) is,

∞
X (2n − 1)πx (2n − 1)cπt
u(x, t) = a sin cos .
n
2L 2L
n=1

Exercise 160. Find the modes of oscillation and their frequencies for a rectangular
drum head of width a and height b. The modes of oscillation are eigensolutions of

2
u = c ∆u, 0 < x < a, 0 < y < b,
tt
u(0, y) = u(a, y) = u(x, 0) = u(x, b) = 0.

Solution:

2
u = c ∆u, 0 < x < a, 0 < y < b, (8.1)
tt
u(0, y) = u(a, y) = u(x, 0) = u(x, b) = 0.

We substitute the separation of variables u(x, y, t) = X(x)Y (y)T(t) into Equa-
tion 8.1.

T 00 X 00 Y 00
= + = −ν
2
c T X Y
X 00 Y 00
= − − ν = −µ
X Y

49