Page 10 - 6413


 F
E  (2.1)
q
The intensity of the electric field at the given point of space is a
physical quantity that equals the ratio of the force F, with which the

electric field affects the unit, at positive point, tests the charge located
at the given point to the value of test charge q.
The electric field is a vector field and can reveal you what force

charge experiences at a particular position in space. While researching
the electric field around a charge, it is important for the test charge to
be comparatively negligible on the field-producing charge. This will

ensure that the introduction of the test charge does not change the
field-producing charge arrangement. Since the direction of the force
on the charge depends on whether the charge is positive or negative,
this must be specified in a field representation. By convention, a

positive test charge q is used for measuring the field. The SI unit for
N
the electric field is the newton per coulomb   or volt per meter
C
V
 .
m

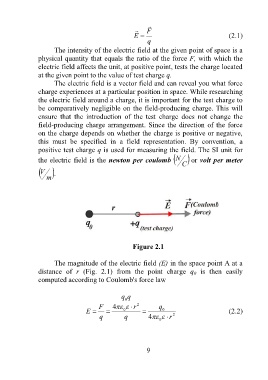
Figure 2.1

The magnitude of the electric field (E) in the space point A at a
distance of r (Fig. 2.1) from the point charge q is then easily
0
computed according to Coulomb's force law

q q
0
F 4 r   2 q
E   0  0 (2.2)
q q 4 r   2
0

9