Page 24 - 6099

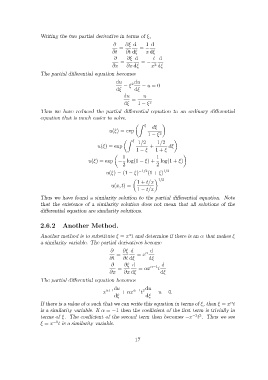
Writing the two partial derivative in terms of ξ,
∂ ∂ξ d 1 d
= =
∂t ∂t dξ x dξ
∂ ∂ξ d t d
= = −
2
∂x ∂x dξ x dξ
The partial differential equation becomes
du 2 du
− ξ − u = 0
dξ dξ
du u
=
dξ 1 − ξ 2
Thus we have reduced the partial differential equation to an ordinary differential
equation that is much easier to solve.
ξ dξ
Z
u(ξ) = exp
1 − ξ 2
Z
ξ 1/2 1/2
u(ξ) = exp + dξ
1 − ξ 1 + ξ

1 1
u(ξ) = exp − log(1 − ξ) + log(1 + ξ)
2 2
u(ξ) = (1 − ξ) −1/2 (1 + ξ) 1/2
1/2
1 + t/x
u(x, t) =
1 − t/x
Thus we have found a similarity solution to the partial differential equation. Note
that the existence of a similarity solution does not mean that all solutions of the
differential equation are similarity solutions.

2.6.2 Another Method.

α
Another method is to substitute ξ = x t and determine if there is an α that makes ξ
a similarity variable. The partial derivatives become
∂ ∂ξ d α d
= = x
∂t ∂t dξ dξ
∂ ∂ξ d α−1 d
= = αx t
∂x ∂x dξ dξ
The partial differential equation becomes
du du
x α+1 + αx α−1 2 − u = 0.
t
dξ dξ
α
If there is a value of α such that we can write this equation in terms of ξ, then ξ = x t
is a similarity variable. If α = −1 then the coefficient of the first term is trivially in
−2 2
terms of ξ. The coefficient of the second term then becomes −x t . Thus we see
−1
ξ = x t is a similarity variable.

17