Page 19 - 6099

Adding these two will give the general solution to the nonhomogeneous equation
1
1
u(ξ, η) = e − ξ f(η) + e 4 1 (3ξ+2η)
4
15
Finally, substituting the expressions for ξ and η in terms of (x, y), we will obtain the
solution
1
1
u(x, y) = e − (2x−4y) f(4x + 2y) + e 4 1 (2x+16y)
4
15

2.4 The variable coefficient equation

The equation
u x + yu y = 0 (2.13)

y

x

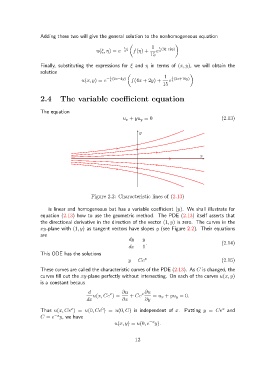
Figure 2.2: Characteristic lines of (2.13)

is linear and homogeneous but has a variable coefficient (y). We shall illustrate for
equation (2.13) how to use the geometric method. The PDE (2.13) itself asserts that
the directional derivative in the direction of the vector (1, y) is zero. The curves in the
xy-plane with (1, y) as tangent vectors have slopes y (see Figure 2.2). Their equations
are
dy y
= . (2.14)
dx 1
This ODE has the solutions
y = Ce x (2.15)
These curves are called the characteristic curves of the PDE (2.13). As C is changed, the
curves fill out the xy-plane perfectly without intersecting. On each of the curves u(x, y)
is a constant becaus
d ∂u ∂u
x
u(x, Ce ) = + Ce x = u x + yu y = 0.
dx ∂x ∂y
x
x
0
Thus u(x, Ce ) = u(0, Ce ) = u(0, C) is independent of x. Putting y = Ce and
−x
C = e y, we have
−x
u(x, y) = u(0, e y).
12