Page 76 - 4749

Q P
   . (4.1)
F F

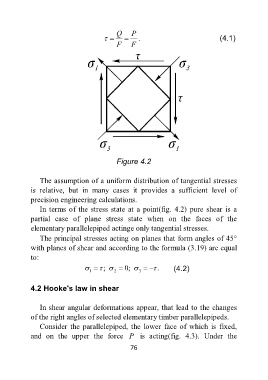
Figure 4.2

The assumption of a uniform distribution of tangential stresses
is relative, but in many cases it provides a sufficient level of
precision engineering calculations.
In terms of the stress state at a point(fig. 4.2) pure shear is a
partial case of plane stress state when on the faces of the
elementary parallelepiped actinge only tangential stresses.
The principal stresses acting on planes that form angles of 45
with planes of shear and according to the formula (3.19) are equal
to:
  ;    0;    (4.2)
. 
1 2 3

4.2 Hooke's law in shear

In shear angular deformations appear, that lead to the changes
of the right angles of selected elementary timber parallelepipeds.
Consider the parallelepiped, the lower face of which is fixed,
and on the upper the force P is acting(fig. 4.3). Under the
76