Page 64 - 4749

3.6 Components deformations. The concept of deformed
state of the point

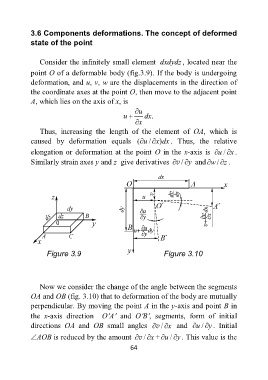
Consider the infinitely small element dxdydz , located near the
point O of a deformable body (fig.3.9). If the body is undergoing
deformation, and u, v, w are the displacements in the direction of
the coordinate axes at the point O, then move to the adjacent point
A, which lies on the axis of x, is
u 
u  dx .
x 
Thus, increasing the length of the element of OA, which is
caused by deformation equals ( u / x )dx . Thus, the relative
elongation or deformation at the point O in the x-axis is u / x .
Similarly strain axes y and z give derivatives v / y and w / z .

Figure 3.9 Figure 3.10

Now we consider the change of the angle between the segments
ОА and ОВ (fig. 3.10) that to deformation of the body are mutually
perpendicular. By moving the point A in the y-axis and point B in
the x-axis direction ОА and ОВ, segments, form of initial
directions OA and ОВ small angles v / x and u / y . Initial
АОВ is reduced by the amount v / x + u / y . This value is the
64