Page 65 - 4749

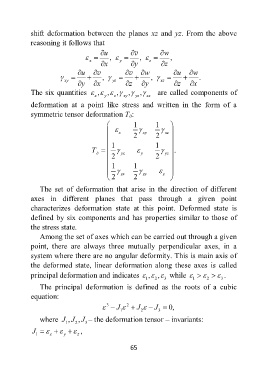
shift deformation between the planes xz and yz. From the above
reasoning it follows that
u  v  w
  ,   ,   ,
x y z
x  y  z 
u  v v  w u   w
   ,    ,    .
xy yz xz
y  x  z  y  z  x 
The six quantities  , , ,  , , are called components of
x y z xy yz xz
deformation at a point like stress and written in the form of a
symmetric tensor deformation Т д:
 1 1 
  x 2  xy 2  xz 
 
Т   1   1   .
д  2 yx y 2 yz 
 
 1  1   
 zx zy z 
 2 2 
The set of deformation that arise in the direction of different
axes in different planes that pass through a given point
characterizes deformation state at this point. Deformed state is
defined by six components and has properties similar to those of
the stress state.
Among the set of axes which can be carried out through a given
point, there are always three mutually perpendicular axes, in a
system where there are no angular deformity. This is main axis of
the deformed state, linear deformation along these axes is called
principal deformation and indicates , ,   while      .
1 2 3 1 2 3
The principal deformation is defined as the roots of a cubic
equation:
2
3
  J   J   J  0,
1 2 3
,
,
where J J J – the deformation tensor – invariants:
1 2 3
J       ,
1 x y z
65