Page 14 - 4638

dt
I
Amount of thermal energy Q  Q    q  F      F  J ,  
dx
where τ - time, c .

The equation states that the heat flow rate q in the x direction is directly

proportional to the heat conduction coefficient λ, temperature gradient dt/dx and the

cross sectional area F normal to the heat flow.

The effectiveness by which heat is transferred through a material (see Figure 5) is

measured by the thermal conductivity, λ. A good conductor, such as copper, has a high

conductivity; a poor conductor, or an insulator, has a low conductivity. Conductivity is

measured in watts per meter per Kelvin (W/mK). In heat transfer, a positive q means

that heat is flowing into the body, and a negative q represents heat leaving the body.

The minus sign in Eqn. 1 indicates that the heat flow is positive in the

direction of decreasing temperature.

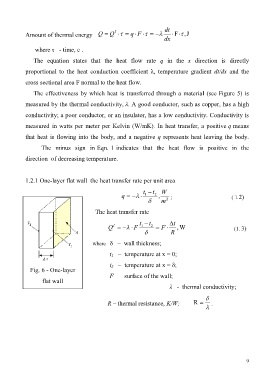
1.2.1 One-layer flat wall the heat transfer rate per unit area

t 1 t  2 W
q     , 2 ; ( 1.2)
 m

The heat transfer rate

t t  t 
I
Q    F 1 2  F  , W (1. 3)
 R

where δ – wall thickness;

t 1 – temperature at x = 0;

t 2 – temperature at x = δ;
Fig. 6 - One-layer
F – surface of the wall;
flat wall
λ - thermal conductivity;


R – thermal resistance, K/W; R  .


9