Page 11 - 4624

b
§·
F F ¨¸ 1-4
y
c
©¹
here the y component is a negative scalar since Fy is directed along the
negative y axis.
It is important to keep in mind that this positive and negative
scalar notation is to be used only for computational purposes, not for
graphical representations in figures. Throughout the book, the head of
a vector arrow in any figure indicates the sense of the vector
graphically; algebraic signs are not used for this purpose. Thus, the
vectors in Figs. 1-1,a and 1-1,b are designated by using boldface
(vector) notation. Whenever italic symbols are written near vector
arrows in figures, they indicate the magnitude of the vector, which is
always a positive quantity.
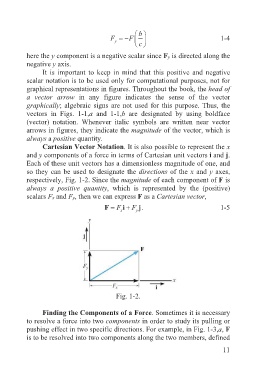
Cartesian Vector Notation. It is also possible to represent the x
and y components of a force in terms of Cartesian unit vectors i and j.
Each of these unit vectors has a dimensionless magnitude of one, and
so they can be used to designate the directions of the x and y axes,
respectively, Fig. 1-2. Since the magnitude of each component of F is
always a positive quantity, which is represented by the (positive)
scalars Fx and Fy, then we can express F as a Cartesian vector,
F F i F j. 1-5
x
y

Fig. 1-2.

Finding the Components of a Force. Sometimes it is necessary
to resolve a force into two components in order to study its pulling or
pushing effect in two specific directions. For example, in Fig. 1-3,a, F
is to be resolved into two components along the two members, defined

11