Page 45 - 4549

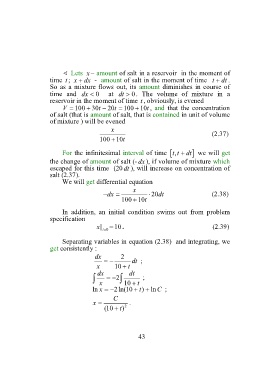
 Lets x – amount of salt in a reservoir in the moment of
time t ; x  dx - amount of salt in the moment of time t  dt .
So as a mixture flows out, its amount diminishes in course of
0
time and dx  at dt  . The volume of mixture in a
0
reservoir in the moment of time t , obviously, is evened
V  100 30t  20t  100 10t , and that the concentration
of salt (that is amount of salt, that is contained in unit of volume
of mixture ) will be evened
x
(2.37)
100 10t

For the infinitesimal interval of time  ,t t  dt  we will get
the change of amount of salt (- dx ), if volume of mixture which
escaped for this time (20 dt ), will increase on concentration of
salt (2.37).
We will get differential equation
x
dx   20dt (2.38)
100 10t

In addition, an initial condition swims out from problem
specification
x|  10 . (2.39)
t 0

Separating variables in equation (2.38) and integrating, we
get consistently :
dx 2
  dt ;
x 10 t
dx dt
   2  ;
x 10 t
ln x   2ln(10 t ) lnC ;
C
x  .
(10 t ) 2

43