Page 102 - 4549

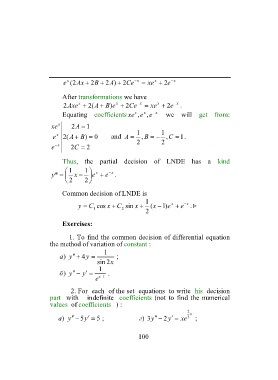
x
x
e 2( Ax  2 B  2 A  2) Ce  x  xe  e 2  x

After transformations we have
x
x
x
2 Axe  (2 A  B) e  2 Ce  x  xe  e 2  x .
x
Equating coefficients xe , e x e ,  x we will get from:
xe x 2 A 1
1 1
x
e ( 2 A  B )  0 and A  , B   ,C  1.
2 2
e x 2 C 2

Thus, the partial decision of LNDE has a kind
 1 1 

x
x
y *  x   e  e .
 2 2 

Common decision of LNDE is
1 x  x
y  C cos x  C sin x  ( x  )1 e  e .
1 2
2

Exercises:

1. To find the common decision of differential equation
the method of variation of constant :
1
)a y  4y  ;
sin 2x
1
)б y  y  .
e x 1

2. For each of the set equations to write his decision
part with indefinite coefficients (not to find the numerical
values of coefficients ) :
2
x
а) y  5y  5 ; г) 3y  2y  xe 3 ;

100