Page 40 - 4498

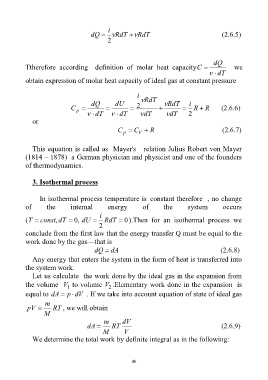
i
dQ   RdT  RdT (2.6.5)
2

dQ
Ttherefore according definition of molar heat capacityC  we
  dT
obtain expression of molar heat capacity of ideal gas at constant pressure

i
 RdT
dQ dU  RdT i
C    2   R  R (2.6.6)
p
  dT   dT  dT  dT 2
or
C  C  R (2.6.7)
p
V

This equation is called as Mayer's relation Julius Robert von Mayer

(1814 – 1878) a German physician and physicist and one of the founders
of thermodynamics.

3. Isothermal process

In isothermal process temperature is constant therefore , no change
of the internal energy of the system occurs

i
(  constT ,dT  , 0 dU  RdT  0).Then for an isothermal process we
2
conclude from the first law that the energy transfer Q must be equal to the
work done by the gas—that is
dQ  dA (2.6.8)

Any energy that enters the system in the form of heat is transferred into
the system work.

Let us calculate the work done by the ideal gas in the expansion from
the volume V to volume V .Elementary work done in the expansion is
1
2
equal to dA  p  dV . If we take into account equation of state of ideal gas
m
pV  RT , we will obtain
M
m dV
dA  RT (2.6.9)
M V
We determine the total work by definite integral as in the following:

40