Page 36 - 4498

i m
U  N A kT (2.2.4)
2 M

2.3 Work Done During Alteration of Gas Volume

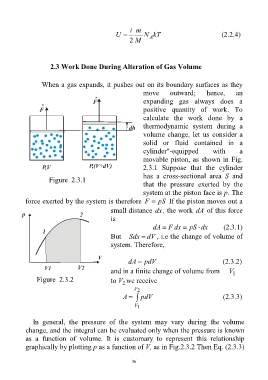
When a gas expands, it pushes out on its boundary surfaces as they
move outward; hence, an
expanding gas always does a

positive quantity of work. To
calculate the work done by a
thermodynamic system during a
volume change, let us consider a

solid or fluid contained in a
cylinder"-equipped with a
movable piston, as shown in Fig.

2.3.1 Suppose that the cylinder
has a cross-sectional area S and
Figure 2.3.1
that the pressure exerted by the

system at the piston face is p. The
force exerted by the system is therefore F  pS If the piston moves out a

small distance dx, the work dA of this force
is
dA  F dx  pS  dx (2.3.1)

But Sdx  dV , i.e the change of volume of
system. Therefore,

dA  pdV (2.3.2)
and in a finite change of volume from V
1
Figure 2.3.2 to V we receive
2
V 2
A  pdV (2.3.3)

V 1

In general, the pressure of the system may vary during the volume

change, and the integral can be evaluated only when the pressure is known
as a function of volume. It is customary to represent this relationship
graphically by plotting p as a function of V, as in Fig.2.3.2 Then Eq. (2.3.3)

36