Page 70 - 4263

Analytic geometry in space

z

− →
Q n
d
(p)
N M 0
− 0 n d
→
. . . . . . . . . . . . . y
0

x

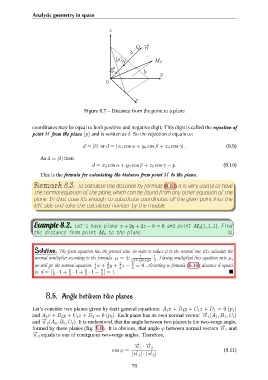
Figure 8.7 – Distance from the point to a plane

coordinates may be equal to both positive and negative digit. This digit is called the rejection of
point M from the plane (p) and is written as δ. So the rejection d equals to:

d = |δ| or d = |x 0 cos α + y 0 cos β + x 0 cos γ| . (8.9)

As d = |δ| then
d = x 0 cos α + y 0 cos β + z 0 cos γ − p. (8.10)

This is the formula for calculating the distance from point M to the plane.

Remark 8.3. To calculate the distance by formula (8.10) it is very useful to have
the normal equation of the plane, which can be found from any other equation of the
plane. In that case it’s enough to substitute coordinates of the given point into the
left side and take the calculated number by the module.

Example 8.2. Let’s have plane x + 2y + 2z − 8 = 0 and point M 0 (1, 1, 1). Find
the distance from point M 0 to the plane. ,

Solution. The given equation has the general view. In order to reduce it to the normal one, let’s calculate the
1 1
normal multiplier according to the formula: µ = ± √ = . Having multiplied this equation onto µ,
2
2
1 +2 +2 2 3
1 2 2 8
we will get the normal equation: x + y + z − = 0. According to formula (8.10), distance d equals
2 2 3 3 3 3
to: d = 1 · 1 + · 1 + · 1 − 8 = 1.
3 3 3 3
8.5. Angle between two planes

Let’s consider two planes given by their general equations: A 1 x + B 1 y + C 1 z + D 1 = 0 (p 1 )
−→
and A 2 x + B 2 y + C 2 z + D 2 = 0 (p 2 ). Each plane has its own normal vector: n 1 (A 1 , B 1 , C 1 )
−→
and n 2 (A 2 , B 2 , C 2 ). It is understood, that the angle between two planes is the two-verge angle,
−→
formed by these planes (fig. 8.8). It is obvious, that angle φ between normal vectors n 1 and
−→
n 2 equals to one of contiguous two-verge angles. Therefore,
−→ −→
n 1 · n 2
cos φ = −→ −→ (8.11)
| n 1 | · | n 2 |

70