Page 71 - 4263

Equations of the straight line in space

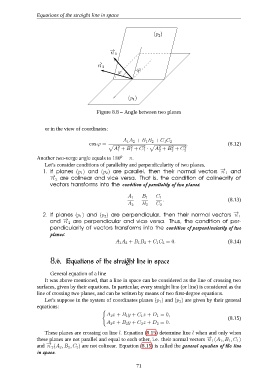
(p 2 )

−→
n 1
−→
n 2
φ
φ

(p 1 )
. . . . . . .
Figure 8.8 – Angle between two planes

or in the view of coordinates:

A 1 A 2 + B 1 B 2 + C 1 C 2
cos φ = √ √ . (8.12)
2 2 2 2 2 2
A + B + C · A + B + C
1 1 1 2 2 2
0
Another two-verge angle equals to 180 − π.
Let’s consider conditions of parallelity and perpendicularity of two planes.
−→
1. If planes (p 1 ) and (p 2 ) are parallel, then their normal vectors n 1 and
−→
n 2 are colinear and vice versa. That is, the condition of colinearity of
vectors transforms into the condition of parallelity of two planes:

A 1 B 1 C 1
= = . (8.13)
A 2 B 2 C 2

−→
2. If planes (p 1 ) and (p 2 ) are perpendicular, then their normal vectors n 1
−→
and n 2 are perpendicular and vice versa. Thus, the condition of per-
pendicularity of vectors transforms into the condition of perpendicularity of two
planes:
A 1 A 2 + B 1 B 2 + C 1 C 2 = 0. (8.14)

8.6. Equations of the straight line in space

General equation of a line
It was above mentioned, that a line in space can be considered as the line of crossing two
surfaces, given by their equations. In particular, every straight line (or line) is considered as the
line of crossing two planes, and can be written by means of two first-degree equations.
Let’s suppose in the system of coordinates planes (p 1 ) and (p 2 ) are given by their general
equations:
{
A 1 x + B 1 y + C 1 z + D 1 = 0,
(8.15)
A 2 x + B 2 y + C 2 z + D 2 = 0.

These planes are crossing on line l. Equation (8.15) determine line l when and only when
− →
these planes are not parallel and equal to each other, i.e. their normal vectors n 1 (A 1 , B 1 , C 1 )
and n 2 (A 2 , B 2 , C 2 ) are not colinear. Equation (8.15) is called the general equation of the line
−→
in space.

71