Page 36 - Microsoft Word - 4236

3.2.2 RECTANGULAR DIMETRY PROJECTION

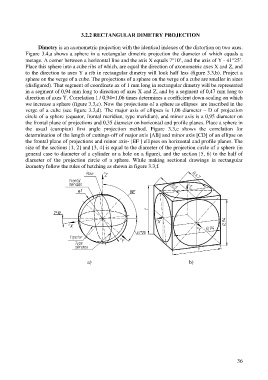
Dimetry is an axonometric projection with the identical indexes of the distortion on two axes.
Figure 3.4,а shows a sphere in a rectangular dimetric projection the diameter of which equals a
metage. A corner between a horizontal line and the axis X equals 710’, and the axis of Y - 4125’.
Place this sphere into a cube ribs of which, are equal the direction of axonometric axes X and Z, and
to the direction to axes Y a rib in rectangular dimetry will look half less (figure 3.3,b). Project a
sphere on the verge of a cube. The projections of a sphere on the verge of a cube are smaller in sizes
(disfigured). That segment of coordinate ax of 1 mm long in rectangular dimetry will be represented
in a segment of 0,94 mm long to direction of axes X and Z, and by a segment of 0,47 mm long to
direction of axes Y. Correlation 1 / 0,94=1,06 times determines a coefficient down-scaling on which
we increase a sphere (figure 3.3,c). Now the projections of a sphere as ellipses are inscribed in the
verge of a cube (see figure 3.3,d). The major axis of ellipses is 1,06 diameter – D of projection
circle of a sphere (equator, frontal meridian, type meridian), and minor axis is a 0,95 diameter on
the frontal plane of projections and 0,35 diameter on horizontal and profile planes. Place a sphere in
the usual (europian) first angle projection method. Figure 3.3,e shows the correlation for
determination of the length of cuttings-off of major axis [AB] and minor axis [CD] of an ellipse on
the frontal plane of projections and minor axis- [EF ] ellipses on horizontal and profile planes. The
size of the sections [1, 2] and [3, 4] is equal to the diameter of the projection circle of a sphere (in
general case to diameter of a cylinder or a hole on a figure), and the section [5, 6] to the half of
diameter of the projection circle of a sphere. While making sectional drawings in rectangular
іzometry follow the rules of hatching as shown in figure 3.3,f.

а) b)

36