Page 54 - 4167

According to the law of conservation of energy, energy cannot be
created or destroyed, hence this energy cannot disappear. Instead, it is
stored as potential energy. If the spring is released or the mass is
dropped, this stored energy will be converted into kinetic energy by the

restoring force, which is elasticity in the case of the spring, and gravity
in the case of the mass. The more formal definition is that potential
energy is the energy difference between the energy of an object in a

given position and its energy at a reference position.
There are various types of potential energy, each associated with
a particular type of force. More specifically, every conservative force
gives rise to potential energy. For example, the work of an elastic force

is called elastic potential energy; work of the gravitational force is called
gravitational potential energy; work of the Coulomb force is called
electric potential energy; work of the strong nuclear force or weak

nuclear force acting on the baryon charge is called nuclear potential
energy; work of intermolecular forces is called intermolecular potential
energy. Chemical potential energy, such as the energy stored in fossil

fuels, is the work of the Coulomb force during rearrangement of mutual
positions of electrons and nuclei in atoms and molecules. Thermal
energy usually has two components: the kinetic energy of random

motions of particles and the potential energy of their mutual positions.
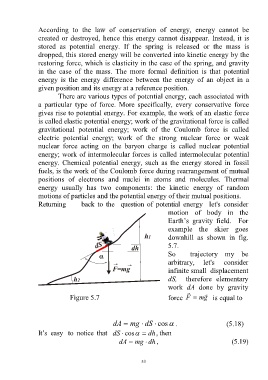
Returning back to the question of potential energy let's consider
motion of body in the
Earth’s gravity field. For

example the skier goes
downhill as shown in fig.
5.7.

So trajectory my be
arbitrary, let's consider
infinite small displacement
dS, therefore elementary

work dA done by gravity
 
Figure 5.7 force F  m g is equal to

dA  mg  dS  cos  . (5.18)

It’s easy to notice that dS  cos   dh, then
dA  mg  dh, (5.19)

53