Page 53 - 4167

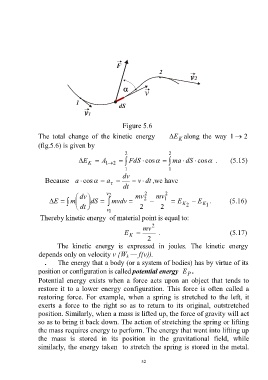
Figure 5.6

The total change of the kinetic energy E K along the way 1 
2
(fig.5.6) is given by
2 2
E K  A 1 2  FdS  cos  ma  dS  cos . (5.15)


1 1
dv
Because a  cos   a   v  dt ,we have

dt
v 2 2 2
 dv  mv 2 mv 1
E   m  dS   mvdv    E K  E K . (5.16)
 dt  v 1 2 2 2 1

Thereby kinetic energy of material point is equal to:
2
mv
E K  . (5.17)
2
The kinetic energy is expressed in joules. The kinetic energy

depends only on velocity v {W — f{v)).
k
. The energy that a body (or a system of bodies) has by virtue of its
position or configuration is called potential energy E .
P
Potential energy exists when a force acts upon an object that tends to
restore it to a lower energy configuration. This force is often called a

restoring force. For example, when a spring is stretched to the left, it
exerts a force to the right so as to return to its original, outstretched
position. Similarly, when a mass is lifted up, the force of gravity will act
so as to bring it back down. The action of stretching the spring or lifting

the mass requires energy to perform. The energy that went into lifting up
the mass is stored in its position in the gravitational field, while
similarly, the energy taken to stretch the spring is stored in the metal.

52