Page 59 - 4167

distorted during the action on the impulsive forces, but they return to their
original shapes and the ball springs away from the plate. In this case the
bodies are only temporary deformed and they regain their original shapes
immediately after the collision. Bodies which return to their original

shapes after the collision are said to be elastic.
The perfectly elastic collision is an impact after which the shapes
of the bodies are completely restored, the movements of bodies are

independent and mechanical energy does not transform into other forms
of energy.

before

collision

after

collision

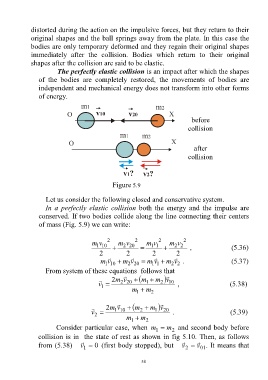
Figure 5.9

Let us consider the following closed and conservative system.

In a perfectly elastic collision both the energy and the impulse are
conserved. If two bodies collide along the line connecting their centers
of mass (Fig. 5.9) we can write:

2 2 2 2
m 1 v 10 m 2 v 20 m 1 v 1 m 2 v 2
   , (5.36)
2  2  2   2

m 1 v  m 2 v 20  m 1 v  m 2 v . (5.37)
1
2
10
From system of these equations follows that


 2m v  m  m v
v  2 20 1 2 10 , (5.38)
1
m  m 2
1

 
 2m v  m  m v
v  1 10 2 1 20 . (5.39)
2
m  m 2
1
Consider particular case, when m  m and second body before
2
1
collision is in the state of rest as shown in fig 5.10. Then, as follows
  
from (5.38) v 1  0 (first body stopped), but v  v . It means that
01
2
58