Page 43 - 4167

Motions of stars and planets have been observed by people for
many centuries.
Kepler's Laws
Kepler deduced three

simple laws of
planetarymotion:
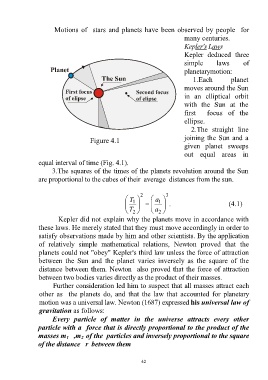
1.Each planet

moves around the Sun
in an elliptical orbit
with the Sun at the
first focus of the

ellipse.
2.The straight line
joining the Sun and a
Figure 4.1
given planet sweeps
out equal areas in
equal interval of time (Fig. 4.1).

3.The squares of the times of the planets revolution around the Sun
are proportional to the cubes of their average distances from the sun.

2 3
 T   a 
 1    1  . (4.1)




 T 2   a 2 
Kepler did not explain why the planets move in accordance with
these laws. He merely stated that they must move accordingly in order to
satisfy observations made by him and other scientists. By the application

of relatively simple mathematical relations, Newton proved that the
planets could not "obey" Kepler's third law unless the force of attraction

between the Sun and the planet varies inversely as the square of the
distance between them. Newton also proved that the force of attraction
between two bodies varies directly as the product of their masses.
Further consideration led him to suspect that all masses attract each

other as the planets do, and that the law that accounted for planetary
motion was a universal law. Newton (1687) expressed his universal law of
gravitation as follows:

Every particle of matter in the universe attracts every other
particle with a force that is directly proportional to the product of the
masses m 1 ,m of the particles and inversely proportional to the square
2
of the distance r between them

42