Page 23 - 6742

mass moment of inertia;  S   і – is velocity of і-th link’s center of
mass;  – is і-th link’s angular velocity; m – is і-th link’s mass.
  і
  і
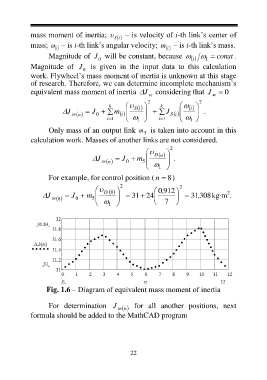
Magnitude of J will be constant, because    i   const .
1
0
Magnitude of J is given in the input data to this calculation
0
work. Flywheel’s mass moment of inertia is unknown at this stage
of research. Therefore, we can determine incomplete mechanism’s
equivalent mass moment of inertia J зв considering that J м  0
k     2 k      2
 зв  J 0  m    S і    J S    i  .


J


i
i
  n
 i 1     i 1   1 
1
Only mass of an output link m is taken into account in this
5
calculation work. Masses of another links are not considered.
  2
J зв   n  J 0  m 5  D   n  .


1 
 
For example, for control position ( n 8)
   8  2  0, 912  2
2
 J зв  J  m  D    31 24   31, 308kg∙m .
5 
0
  8
1 
   7 

Fig. 1.6 – Diagram of equivalent mass moment of inertia

For determination J зв   n for all another positions, next
formula should be added to the MathCAD program

22