Page 44 - 6686

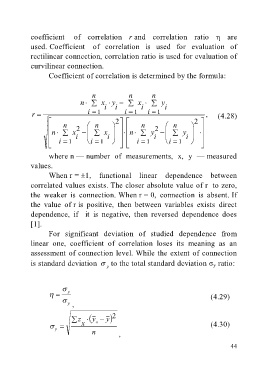
coefficient of correlation r and correlation ratio η are
used. Coefficient of correlation is used for evaluation of
rectilinear connection, correlation ratio is used for evaluation of
curvilinear connection.
Coefficient of correlation is determined by the formula:

n n n
 n  х і  у і   х і   у і
r  i  1 i  1 i  1 , (4.28)
 n  n  2  n  n  2 
  n  х 2    x      n  y 2    y   
 і  i    і  i  
 i  1  i 1    i  1  i 1  





where n — number of measurements, x, y — measured
values.
When r = ±1, functional linear dependence between
correlated values exists. The closer absolute value of r to zero,
the weaker is connection. When r = 0, connection is absent. If
the value of r is positive, then between variables exists direct
dependence, if it is negative, then reversed dependence does
[1].
For significant deviation of studied dependence from
linear one, coefficient of correlation loses its meaning as an
assessment of connection level. While the extent of connection
is standard deviation  to the total standard deviation σ y ratio:
y


  y (4.29)

y ,
2
 z  y   y
x
  x (4.30)
y
n ,
44