Page 20 - 6686

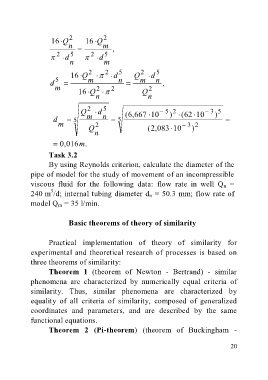
2 2
16 Q 16 Q
n m
 ,
2 5 2 5
  d   d
n m
2 2 5 2 5
16 Q   d Q d 
5 m n m n
d   ,
m 2 2 2
16 Q   Q
n n
2 5
Q d  , 6 ( 667  10  5 ) 2 (  62  10  3 ) 5
d  5 m n  5 
m 2  3 2
Q , 2 ( 083  10 )
n
 , 0 016m .
Task 3.2
By using Reynolds criterion, calculate the diameter of the
pipe of model for the study of movement of an incompressible
viscous fluid for the following data: flow rate in well Q n =
3
240 m /d; internal tubing diameter d n = 50.3 mm; flow rate of
model Q m = 35 l/min.

Basic theorems of theory of similarity

Practical implementation of theory of similarity for
experimental and theoretical research of processes is based on
three theorems of similarity:
Theorem 1 (theorem of Newton - Bertrand) - similar
phenomena are characterized by numerically equal criteria of
similarity. Thus, similar phenomena are characterized by
equality of all criteria of similarity, composed of generalized
coordinates and parameters, and are described by the same
functional equations.
Theorem 2 (Pi-theorem) (theorem of Buckingham -

20