Page 39 - 6685

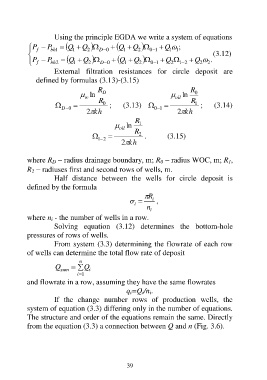
Using the principle EGDA we write a system of equations
P  P bh 1  Q 1  Q 2  D 0  Q 1  Q 2  0 1  Q 1 1 ;
 f
 (3.12)
P  P bh 2  Q 1  Q 2  D 0  Q 1  Q 2  0 1  Q 2  1 2 Q 2  2 .
 f
External filtration resistances for circle deposit are
defined by formulas (3.13)-(3.15)
R R
 ln D  ln 0
oil
w
 D 0  R 0 ; (3.13)  0  R 1 ; (3.14)
2  kh 1  2 kh
R
 ln 1
oil
 1  R 2 . (3.15)
2
 2 kh

where R D  radius drainage boundary, m; R 0  radius WOC, m; R 1,
R 2  radiuses first and second rows of wells, m.
Half distance between the wells for circle deposit is
defined by the formula
 R
  i ,
i
n i
where n i - the number of wells in a row.
Solving equation (3.12) determines the bottom-hole
pressures of rows of wells.
From system (3.3) determining the flowrate of each row
of wells can determine the total flow rate of deposit
n
Q sum  Q
i
 i 1
and flowrate in a row, assuming they have the same flowrates
q i=Q i/n i.
If the change number rows of production wells, the
system of equation (3.3) differing only in the number of equations.
The structure and order of the equations remain the same. Directly
from the equation (3.3) a connection between Q and n (Fig. 3.6).

39