Page 82 - 6637

Supplementary Problems

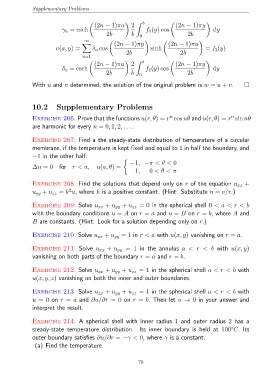
Z b
(2n − 1)πa 2 (2n − 1)πy
γ = csch f (y) cos dy
n
1
2b b 0 2b
∞
(2n − 1)πy (2n − 1)πa
X
v(a, y) = δ cos sinh = f (y)
n
2
2b 2b
n=1
Z b
(2n − 1)πa 2 (2n − 1)πy
δ = csch f (y) cos dy
n
2
2b b 0 2b
With u and v determined, the solution of the original problem is w = u + v.

10.2 Supplementary Problems

n
n
Exercise 206. Prove that the functions u(r, θ) = r cos nθ and u(r, θ) = r sin nθ
are harmonic for every n = 0, 1, 2, . . . .

Exercise 207. Find a the steady-state distribution of temperature of a circular
membrane, if the temperature is kept fixed and equal to 1 in half the boundary, and
−1 in the other half:

−1, −π < θ < 0
∆u = 0 for r < a, u(a, θ) =
1, 0 < θ < π

Exercise 208. Find the solutions that depend only on r of the equation u xx +
2
u + u zz = k u, where k is a positive constant. (Hint: Substitute u = v/r.)
yy
Exercise 209. Solve u xx + u yy + u zz = 0 in the spherical shell 0 < a < r < b
with the boundary conditions u = A on r = a and u = B on r = b, where A and

B are constants. (Hint: Look for a solution depending only on r.)

Exercise 210. Solve u xx + u yy = 1 in r < a with u(x, y) vanishing on r = a.

Exercise 211. Solve u xx + u yy = 1 in the annulus a < r < b with u(x, y)
vanishing on both parts of the boundary r = a and r = b.

Exercise 212. Solve u xx + u yy + u zz = 1 in the spherical shell a < r < b with
u(x, y, z) vanishing on both the inner and outer boundaries.

Exercise 213. Solve u xx + u yy + u zz = 1 in the spherical shell a < r < b with
u = 0 on r = a and ∂u/∂r = 0 on r = b. Then let a → 0 in your answer and
interpret the result.

Exercise 214. A spherical shell with inner radius 1 and outer radius 2 has a
◦
steady-state temperature distribution. Its inner boundary is held at 100 C. Its
outer boundary satisfies ∂u/∂r = −γ < 0, where γ is a constant.
(a) Find the temperature.

78