Page 77 - 6637

Solved Problems

√
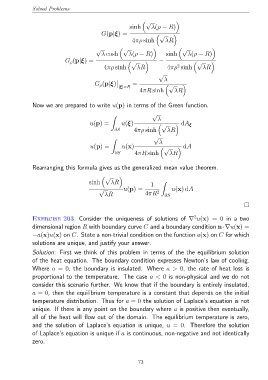
sinh λ(ρ − R)
G(p|ξ) = √
4πρ sinh λR
√ √ √
λ cosh λ(ρ − R) sinh λ(ρ − R)
G (p|ξ) = √ − √
ρ
2
4πρ sinh λR 4πρ sinh λR
√
λ

G (p|ξ) |ξ|=R = √
ρ
4πR sinh λR

Now we are prepared to write u(p) in terms of the Green function.

√
Z
λ
u(p) = u(ξ) √ dA ξ
∂S 4πρ sinh λR
√
Z
λ
u(p) = u(x) √ dA
∂S 4πR sinh λR

Rearranging this formula gives us the generalized mean value theorem.

√
sinh λR 1 Z
√ u(p) = u(x) dA
λR 4πR 2 ∂S

2
Exercise 203. Consider the uniqueness of solutions of ∇ u(x) = 0 in a two
dimensional region R with boundary curve C and a boundary condition n·∇u(x) =
−a(x)u(x) on C. State a non-trivial condition on the function a(x) on C for which

solutions are unique, and justify your answer.
Solution: First we think of this problem in terms of the the equilibrium solution
of the heat equation. The boundary condition expresses Newton’s law of cooling.

Where a = 0, the boundary is insulated. Where a > 0, the rate of heat loss is
proportional to the temperature. The case a < 0 is non-physical and we do not
consider this scenario further. We know that if the boundary is entirely insulated,
a = 0, then the equilibrium temperature is a constant that depends on the initial

temperature distribution. Thus for a = 0 the solution of Laplace’s equation is not
unique. If there is any point on the boundary where a is positive then eventually,
all of the heat will flow out of the domain. The equilibrium temperature is zero,
and the solution of Laplace’s equation is unique, u = 0. Therefore the solution

of Laplace’s equation is unique if a is continuous, non-negative and not identically
zero.

73