Page 20 - 6589

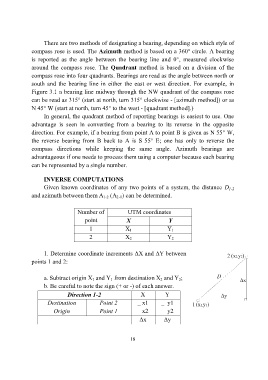
There are two methods of designating a bearing, depending on which style of

compass rose is used. The Azimuth method is based on a 360° circle. A bearing
is reported as the angle between the bearing line and 0°, measured clockwise
around the compass rose. The Quadrant method is based on a division of the
compass rose into four quadrants. Bearings are read as the angle between north or
south and the bearing line in either the east or west direction. For example, in

Figure 3.1 a bearing line midway through the NW quadrant of the compass rose
can be read as 315° (start at north, turn 315° clockwise - [azimuth method]) or as
N 45° W (start at north, turn 45° to the west - [quadrant method].)

In general, the quadrant method of reporting bearings is easiest to use. One
advantage is seen in converting from a bearing to its reverse in the opposite
direction. For example, if a bearing from point A to point B is given as N 55° W,
the reverse bearing from B back to A is S 55° E; one has only to reverse the

compass directions while keeping the same angle. Azimuth bearings are
advantageous if one needs to process them using a computer because each bearing
can be represented by a single number.

INVERSE COMPUTATIONS
Given known coordinates of any two points of a system, the distance D 1-2

and azimuth between them A 1-2 (A 2-1) can be determined.

Number of UTM coordinates
point X Y

1 X 1 Y 1
2 X 2 Y 2

1. Determine coordinate increments ΔX and ΔY between
points 1 and 2:

a. Subtract origin X 1 and Y 1 from destination X 2 and Y 2;

b. Be careful to note the sign (+ or -) of each answer.
Direction 1-2 X Y
Destination Point 2 _ x1 _ y1

Origin Point 1 x2 y2
Δx Δy

18