Page 89 - 6099

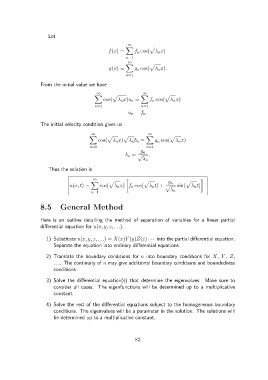
Let
∞
X p
f(x) = f n cos( λ n x)
n=1
∞
X p
g(x) = g n cos( λ n x).
n=1

From the initial value we have
∞ ∞
X p X p
cos( λ n x)a n = f n cos( λ n x)
n=1 n=1
a n = f n .

The initial velocity condition gives us

∞ ∞
X p p X p
cos( λ n x) λ n b n = g n cos( λ n x)
n=1 n=1
g n
b n = √ .
λ n
Thus the solution is

∞
X p p p
g n
u(x, t) = cos( λ n x) f n cos( λ n t) + √ sin( λ n t) .
λ n
n=1
8.5 General Method

Here is an outline detailing the method of separation of variables for a linear partial
differential equation for u(x, y, z, . . .).

1) Substitute u(x, y, z, . . .) = X(x)Y (y)Z(z) · · · into the partial differential equation.
Separate the equation into ordinary differential equations.

2) Translate the boundary conditions for u into boundary conditions for X, Y , Z,
. . .. The continuity of u may give additional boundary conditions and boundedness
conditions.

3) Solve the differential equation(s) that determine the eigenvalues. Make sure to
consider all cases. The eigenfunctions will be determined up to a multiplicative
constant.

4) Solve the rest of the differential equations subject to the homogeneous boundary
conditions. The eigenvalues will be a parameter in the solution. The solutions will
be determined up to a multiplicative constant.

82