Page 62 - 6099

6.6 Waves with a source

The purpose of this section is to solve

2
u tt − c u xx = f(x, t), (6.10)
on the whole line, together with the usual initial conditions

u(x, 0) = φ(x),
u t (x, 0) = ψ(x). (6.11)

where f(x, t) is a given function. For instance, f(x, t) could be interpreted as an external
force acting on an infinitely long vibrating string.
2 2
2
Because L = ∂ −c ∂ is a linear operator, the solution will be the sum of three terms,
t
x
one for φ, one for ψ, and one for f. The first two terms are given already in previous
sections and we must find the third term. We’ll derive the following formula.
Theorem 6.6.1 The unique solution of (6.10), (6.11) is

Z x+ct ZZ
1 1 1
u(x, t) = (φ(x + ct) + φ(x − ct)) + φ + f (6.12)
2 2c x−ct 2c ∆

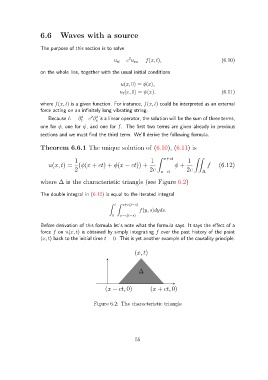
where ∆ is the characteristic triangle (see Figure 6.2)

The double integral in (6.12) is equal to the iterated integral

t
Z Z x+c(t−s)
f(y, s)dyds.
0 x−c(t−s)

Before derivation of this formula let’s note what the formula says. It says the effect of a
force f on u(x, t) is obtained by simply integrating f over the past history of the point
(x, t) back to the initial time t = 0. This is yet another example of the causality principle.

(x, t)

∆

(x − ct, 0) (x + ct, 0)

Figure 6.2: The characteristic triangle

55