Page 58 - 6099

b t = 0
u

b/2

x
-a a
b/2 t = a t = a
u 2c b/2 u c

x x
-3a/2 3a/2 -2a 2a

b/2 t = 2a
c
u
x
-3a -a a 3a

b/2 t = 3a
c
u
x
-4a -2a 2a 4a

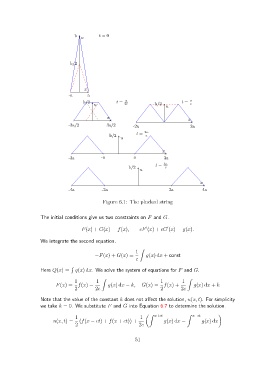
Figure 6.1: The plucked string

The initial conditions give us two constraints on F and G.

0
0
F(x) + G(x) = f(x), −cF (x) + cG (x) = g(x).
We integrate the second equation.

Z
1
−F(x) + G(x) = g(x) dx + const
c
R
Here Q(x) = q(x) dx. We solve the system of equations for F and G.
Z Z
1 1 1 1
F(x) = f(x) − g(x) dx − k, G(x) = f(x) + g(x) dx + k
2 2c 2 2c
Note that the value of the constant k does not affect the solution, u(x, t). For simplicity
we take k = 0. We substitute F and G into Equation 6.7 to determine the solution.

Z x+ct Z x−ct
1 1
u(x, t) = (f(x − ct) + f(x + ct)) + g(x) dx − g(x) dx
2 2c

51