Page 140 - 4624

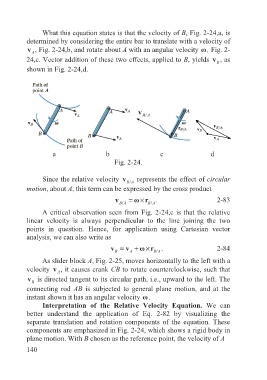
What this equation states is that the velocity of B, Fig. 2-24,a, is
determined by considering the entire bar to translate with a velocity of
v , Fig. 2-24,b, and rotate about A with an angular velocity ω, Fig. 2-
A
24,c. Vector addition of these two effects, applied to B, yields v , as
B
shown in Fig. 2-24,d.

v / B A

a b c d
Fig. 2-24.

Since the relative velocity v / BA represents the effect of circular
motion, about A, this term can be expressed by the cross product
v / BA = ω r / B A . 2-83
×
A critical observation seen from Fig. 2-24,c is that the relative
linear velocity is always perpendicular to the line joining the two
points in question. Hence, for application using Cartesian vector
analysis, we can also write as
×
v B = v A + ω r / BA . 2-84
As slider block A, Fig. 2-25, moves horizontally to the left with a
velocity v , it causes crank CB to rotate counterclockwise, such that
A
v is directed tangent to its circular path, i.e., upward to the left. The
B
connecting rod AB is subjected to general plane motion, and at the
instant shown it has an angular velocity ω.
Interpretation of the Relative Velocity Equation. We can
better understand the application of Eq. 2-82 by visualizing the
separate translation and rotation components of the equation. These
components are emphasized in Fig. 2-24, which shows a rigid body in
plane motion. With B chosen as the reference point, the velocity of A

140