Page 136 - 4624

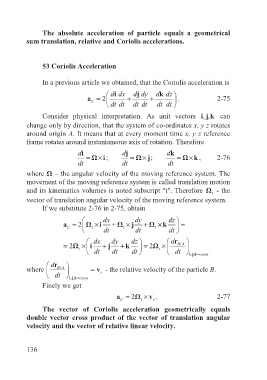
The absolute acceleration of particle equals a geometrical
sum translation, relative and Coriolis accelerations.

53 Coriolis Acceleration

In a previous article we obtained, that the Coriolis acceleration is
j
a = 2   d dx + d dy + d dz  i k  . 2-75
 C dt dt dt dt dt dt 
Consider physical interpretation. As unit vectors ,,i jk can
change only by direction, that the system of co-ordinates x, y z rotates
around origin A. It means that at every moment time x, y z reference
frame rotates around instantaneous axis of rotation. Therefore
di = Ω i; dj = Ω j; dk = Ω k , 2-76
×
×
×
dt dt dt
where Ω – the angular velocity of the moving reference system. The
movement of the moving reference system is called translation motion
and its kinematics volumes is noted subscript "t". Therefore Ω - the
t
vector of translation angular velocity of the moving reference system.
If we substitute 2-76 in 2-75, obtain
 dx dy dz 
a C = 2 Ω t ×i + Ω t × j + Ω t ×k  =

 dt dt dt 
 dx dy dz   dr 
= 2Ω t  × i + j + k  = 2Ω t  × / BA 
 dt dt dt   dt  i ,, =jk const

 dr 
where  / BA  = v - the relative velocity of the particle B.
 dt  i ,, =jk const r
Finely we get
a C = 2Ω t × v . 2-77
r
The vector of Coriolis acceleration geometrically equals
double vector cross product of the vector of translation angular
velocity and the vector of relative linear velocity.

136