Page 53 - 4549

1
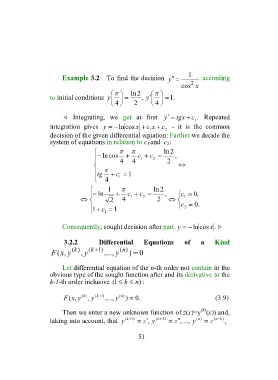
Example 3.2 To find the decision y   according
2
cos x
  ln 2  
to initial conditions  y   ,  y    . 1
 4  2  4 


 Integrating, we get at first y  tgx  c . Repeated
1
integration gives y   ln cos x  c x  c - it is the common
1 2
decision of the given differential equation: Farther we decide the
system of equations in relation to с 1 and с 2:
   ln 2
  ln cos 4  4 c 1  c 2  2 ,

 
 tg   c  1
 4 1

 1  ln 2
c
  ln  c 1  c 2  ,  1  ,0
  2 4 2  
c
  2  .0
 1  c 1  1

Consequently, sought decision after part y   ln cos x .

3.2.2 Differential Equations of a Kind
F (x , y (k ) , y ( k ) 1 ,..., y (n ) )  0

Let differential equation of the n-th order not contain in the
obvious type of the sought function after and its derivative to the
k-1-th order inclusive 1(  k  : ) n

F (x , y (k ) , y ( k ) 1 ,..., y (n ) )  . 0 (3.9)

(k)
Then we enter a new unknown function of z(x)=y (x)) and,
taking into account, that y (k ) 1   z , y (k  ) 2  z ,  ..., y (n )  z (n ) k ,

51