Page 12 - 4549

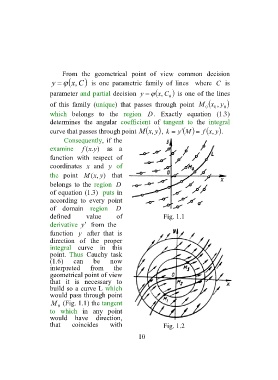
From the geometrical point of view common decision
y   Cx,  is one parametric family of lines where C is
parameter and partial decision y    , Cx  is one of the lines
0
of this family (unique) that passes through point M 0  , yx 0 0 
which belongs to the region D . Exactly equation (1.3)
determines the angular coefficient of tangent to the integral
curve that passes through point  yxM , , k  y    f  yx, .
M
Consequently, if the
examine f (x .y ) as a
function with respect of
coordinates x and y of
the point M (x , ) y that
belongs to the region D
of equation (1.3) puts in
according to every point
of domain region D
defined value of Fig. 1.1
derivative y from the
function y after that is
direction of the proper
integral curve in this
point. Thus Cauchy task
(1.6) can be now
interpreted from the
geometrical point of view
that it is necessary to
build so a curve L which
would pass through point
M (Fig. 1.1) the tangent
0
to which in any point
would have direction,
that coincides with Fig. 1.2

10