Page 114 - 4549

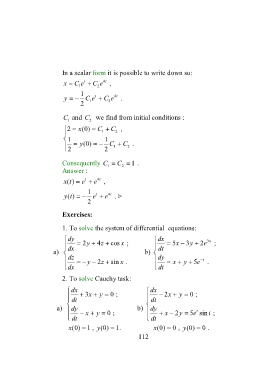
In a scalar form it is possible to write down so:
t
x  C e  C e 4t ,
1 2
1 t 4t
y   C e  C e .
2
1
2
C and C we find from initial conditions :
1 2
 2  x (0)  C  C 2 ,
1

 1 1
  y (0)   C  C 2 .
1
 2 2
Consequently C  C  1 .
1 2
Answer :
t
x ( )t  e  e 4t ,
1 t 4t
y ( )t   e  e . 
2
Exercises:

1. To solve the system of differential equations:

 dy  dx 3x
 dx  2y  4z  cos x ;  dt  5x  3y  2e ;


а)  b) 
 dz   y  2z  sin .x  dy  x  y  5e t  .
  dx   dt

2. To solve Cauchy task:
 dx  dx
 dt  3x  y  0 ;  dt  2x  y  0 ;


 
a) dy b) dy
  x  y  0 ;   x  2y  5 sin ;e t t
  dt   dt
x (0) 1 , (0) 1.y  x (0)  0 , (0)y  0 .
112