Page 30 - 4234

4.5 THE PRINCIPAL LINES OF A PLANE

The lines of a plane which are parallel to the planes of projections are the principal lines of
the plane.
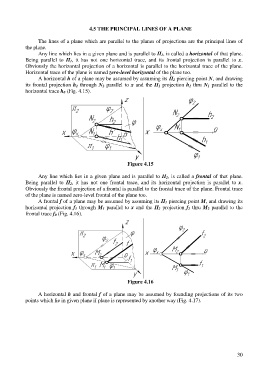
Any line which lies in a given plane and is parallel to Π 1, is called a horizontal of that plane.
Being parallel to Π 1, it has not one horizontal trace, and its frontal projection is parallel to x.
Obviously the horizontal projection of a horizontal is parallel to the horizontal trace of the plane.
Horizontal trace of the plane is named zero-level horizontal of the plane too.
A horizontal h of a plane may be assumed by assuming its Π 2 piercing point N, and drawing
its frontal projection h 2 through N 2 parallel to x and the Π 1 projection h 1 thru N 1 parallel to the
horizontal trace h 0 (Fig. 4.15).

Figure 4.15

Any line which lies in a given plane and is parallel to Π 2, is called a frontal of that plane.
Being parallel to Π 2, it has not one frontal trace, and its horizontal projection is parallel to x.
Obviously the frontal projection of a frontal is parallel to the frontal trace of the plane. Frontal trace
of the plane is named zero-level frontal of the plane too.
A frontal f of a plane may be assumed by assuming its Π 1 piercing point M, and drawing its
horizontal projection f 1 through M 1 parallel to x and the Π 2 projection f 2 thru M 2 parallel to the
frontal trace f 0 (Fig. 4.16).

Figure 4.16

A horizontal h and frontal f of a plane may be assumed by founding projections of its two
points which lie in given plane if plane is represented by another way (Fig. 4.17).

30