Page 28 - 4234

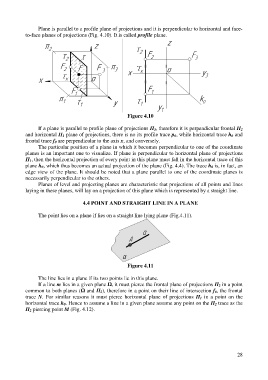
Plane is parallel to a profile plane of projections and it is perpendicular to horizontal and face-
to-face planes of projections (Fig. 4.10). It is called profile plane.

Figure 4.10

If a plane is parallel to profile plane of projections Π 3, therefore it is perpendicular frontal Π 2
and horizontal Π 1 plane of projections, there is no its profile trace p 0, while horizontal trace h 0 and
frontal trace f 0 are perpendicular to the axis x, and conversely.
The particular position of a plane in which it becomes perpendicular to one of the coordinate
planes is an important one to visualize. If plane is perpendicular to horizontal plane of projections
Π 1, then the horizontal projection of every point in this plane must fall in the horizontal trace of this
plane h 0, which thus becomes an actual projection of the plane (Fig. 4.4). The trace h 0 is, in fact, an
edge view of the plane. It should be noted that a plane parallel to one of the coordinate planes is
necessarily perpendicular to the others.
Planes of level and projecting planes are characteristic that projections of all points and lines
laying in these planes, will lay on a projection of this plane which is represented by a straight line.

4.4 POINT AND STRAIGHT LINE IN A PLANE

The point lies on a plane if lies on a straight line lying plane (Fig.4.11).

Figure 4.11

The line lies in a plane if its two points lie in this plane.
If a line m lies in a given plane Ω, it must pierce the frontal plane of projections Π 2 in a point
common to both planes (Ω and Π 2), therefore in a point on their line of intersection f 0, the frontal
trace N. For similar reasons it must pierce horizontal plane of projections Π 1 in a point on the
horizontal trace h 0. Hence to assume a line in a given plane assume any point on the Π 2 trace as the
Π 2 piercing point M (Fig. 4.12).

28